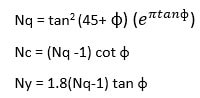Name of the experiment: Angularity number of coarse aggregate test
Aim
of the experiment: To determine the
Angularity number of a given coarse aggregate sample.
Referred Standards:
IS : 2386 (Part I) – 1963 : Indian
standard methods of test for aggregates for concrete particle size and shape.
Summary of test method:
- The
aggregate was compacted in three layers, each layer were given 100 blows
using the standard tamping rod at a rate of 2 blows/second by lifting the
rod 5 cm above the surface of the aggregate and then allowing it to fall
freely.
- After
compacting the third layer, the cylinder was filled to overflowing and
excess material was removed off with temping rod as a straight.
- The
aggregate with cylinder was then weighed. Three separate determinations were
made and mean weight of the aggregate in the cylinder was calculated.
Significance and use:
Degree of packing of particles of one
size depends on their shape. The angularity of aggregates can be estimated from
the proportion of voids among particles compacted in a standard manner. Rounded
aggregates results to minimum void content and angular aggregates results into
more voids. Void in a aggregate system depends on the shape of the aggregate. All
other shapes except rounded aggregates result into more voids. This concept is
utilized in determining the shape of the aggregate by indirect method i.e.
Angularity Number .Angularity number is 67- % of solid volume in a vessel
filled with aggregates in a standard manner. The number 67 represents solid
volume of most rounded gravel.
Observations and Calculations:
Where,
W
= mean weight of the aggregate filling cylinder.
C
= Weight of water required to completely fill the cylinder (i.e. Volume of
cylinder)
Gs =
Specific Gravity of the aggregate
|
||||||||||||||||||||||||||||||||
Results:
The Angularity
number of given sample is given by 9.077
As obtained value is in the range of 8-11 so there is no problem in using these aggregates in pavement design with binders.